I've been going through Landau-Lifshitz textbooks and noticed some tough integrals found in Landau-Lifshitz Mechanics, subsection 14 on Integration of the Equations of Motion. I've managed to complete a couple of them and I thought I'd put them here for records.
I'm looking to solve an integral of them form:
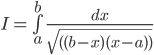
This is a special case where the limits are the roots of the radicand. If we make the transformation:
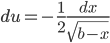
We can now use this to replace one of the factors in the denominator:
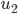and 
are found by the transformation equation, which we can also use to get rid of the 
in the denominator. I'll find them below, but leave them as 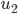and for simplicity.
Now since:
Substituting back into our integral:
Now another transformation can be made, which most conveniently can be chosen as:
Using this transformation on our integral we obtain:
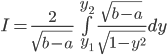
This integral is a standard integral for 
, which we can derive by making the transformation:
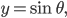
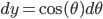
Back into our integral we have:
Since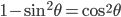 
. This is now a trivial integral, which is just the difference of the limits 
and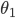 
. The integral becomes:
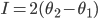
The task now is to find the values and 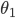by transforming back to the original equation. The 
limits are given by:
The 
limits are therefore:
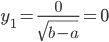
and finally:
Which gives us the result:
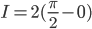
This holds for all 
such that the limits are roots corresponding to the precise shape of 
given at the start. It's kind of amazing that it always comes out to 
, a result which has been in the back of my mind since I first did this integral about a month ago.